We are given an audio file containing music, a Musescore sheet music file, a midi file, and several hints. Of the hints, most importantly:
If you don’t know music, it’s enough to know these concepts:
- Major scale: https://en.wikipedia.org/wiki/G_major
- How rhythm works, such as https://en.wikipedia.org/wiki/Eighth_note
The challenge is tagged as a “stegano” but it’s actually very different from an image stegano - the point of an image stegano is to hide information in a way that does not affect the appearance of the image; this music stegano would sound different if the flag’s contents were changed.
The flag format is AOTW{} and that wrapper part is embedded in the music as well.
Chromatic Scale (12 Notes):
C, C#, D, D#, E, F, F#, G, G#, A, A#, B
Major Scales follow the format:
W W H W W W
Where W is a whole step (skip a note in chromatic scale), and H is a half step (go directly to next note in chromatic scale)
Major (and minor) scales have 7 notes.
G Major Scale (7 Notes)
G, A, B, C, D, E, and F♯
Maybe we can treat notes in the G Major Scale as base 7, starting with G = 0
| C | C# | D | D# | E | F | F# | G | G# | A | A# | B | C | C# | D | D# | E | F | F# |
| C | D | E | F# | G | A | B | C | D | E | F# | ||||||||
| 3 | 4 | 5 | 6 | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
Musical notation for note length/rhythm divisions:
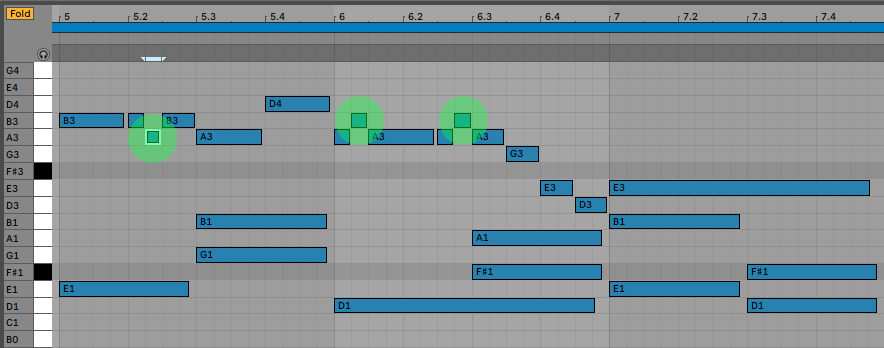
After listening to the song a few times, I noticed that the rhythm occasionally sped up. Where the musical notes normally lined up on evenly divisible eighth/sixteenth notes, there were a small number of odd-numbered sixteenth notes (highlighted).
Reading sheet music is a bit rough for me, so I opened the midi file in Ableton Live and turned on Piano Roll Folding. The extra sixteenth notes I suspected were data bits are highlighted.
Since the hints told us "AOTW{" is encoded in the music, I decided to try to reproduce the encoding in python to verify my guess about the rhythm.
flag = split("AOTW{")
def split(word):
return [char for char in word]
flag = split(flag)['A', 'O', 'T', 'W', '{']
def char_to_dec(char_list):
final = []
for char in char_list:
final.append(ord(char))
return final
decimal = char_to_dec(flag)[65, 79, 84, 87, 123]
It's important to note it required 3 base 7 digits for ascii values. When decoding later, make sure to use 3 digits when calculating the decimal value.
def dec_to_sept(num_list):
final = []
for num in num_list:
base = 7
sept = ''
while num > 0:
sept = str(num % base) + sept
num = num // base
final.append(sept)
return final
septenary = dec_to_sept(decimal)['122', '142', '150', '153', '234']
gmaj = {0: 'G', 1: 'A', 2: 'B', 3: 'C', 4: 'D', 5: 'E', 6: 'F#'}
def sept_to_gmaj(num_list):
final = []
for num in num_list:
for n in split(str(num)):
final.append(gmaj.get(int(n)))
return final
notes = sept_to_gmaj(septenary)['A', 'B', 'B', 'A', 'D', 'B', 'A', 'E', 'G', 'A', 'E', 'C', 'B', 'C', 'D']
After walking through the "data notes" identified manually, I was able to confirm this encoding method produced the same result as the provided music.
On my initial solve, I manually filtered the midi file to contain only "data notes". After solving for points, I went back and wrote a function to select the off-beat data notes from the midi file.
Midi files contain a series of messages (instructions).
{'type': 'time_signature', 'numerator': 4, 'denominator': 4, 'clocks_per_click': 24, 'notated_32nd_notes_per_beat': 8, 'time': 0}
{'type': 'key_signature', 'key': 'G', 'time': 0}
{'type': 'set_tempo', 'tempo': 545455, 'time': 0}
{'type': 'note_on', 'time': 0, 'channel': 1, 'note': 40, 'velocity': 80}
{'type': 'note_on', 'time': 227, 'channel': 0, 'note': 71, 'velocity': 0}
{'type': 'note_on', 'time': 253, 'channel': 0, 'note': 69, 'velocity': 80}
{'type': 'note_on', 'time': 227, 'channel': 0, 'note': 69, 'velocity': 0}
There are many midi message types: time_signature, key_signature, set_tempo, *_change, note_on, note_off, etc.
All midi messages have a time value, which is the number of ticks since the last message.
note_on messages have:
| time | channel | note | velocity |
|---|---|---|---|
| ticks since last message | which voice/instrument | integer value of note on chromatic scale | force/loudness |
We only care about notes with an audible velocity, so anything over 0.
We can calculate the musical note from the integer value with:
chromatic_scale = {0: 'C', 1: 'C#', 2: 'D', 3: 'D#', 4: 'E', 5: 'F', 6: 'F#', 7: 'G', 8: 'G#', 9: 'A', 10: 'A#', 11: 'B'}
chromatic_scale.get(note_integer % 12)We'll need to keep track of our play time since the beginning of the file.
We need to know how many ticks per beat so we can determine the sixteenth note divisions. With the mido library, we can determine how many ticks per beat (quarter note) using:
midi_file = MidiFile('Stegno.mid')
ticks_per_beat = midi_file.ticks_per_beat480
Now we can determine if it's an even or odd sixteenth note with:
sixteenth = (play_time % ticks_per_beat)/(ticks_per_beat/4)
if (sixteenth % 2) != 0:
print("Odd numbered sixteenth note")The full function for selecting notes is below:
chromatic_scale = {0: 'C', 1: 'C#', 2: 'D', 3: 'D#', 4: 'E', 5: 'F', 6: 'F#', 7: 'G', 8: 'G#', 9: 'A', 10: 'A#', 11: 'B'}
def select_notes_from_file(midi_file):
midi_file = MidiFile(midi_file)
track = mido.merge_tracks(midi_file.tracks)
ticks_per_beat = midi_file.ticks_per_beat
notes = []
play_time = 0
for msg in track:
m = msg.dict()
play_time += m.get('time') # Count ticks since beginning of file
# each beat is a quarter note, we care about sixteenth notes
sixteenth = (play_time % ticks_per_beat)/(ticks_per_beat/4)
if (m.get('type') == 'note_on'
and m.get('velocity') != 0
and (sixteenth % 2)): # Select only odd-numbered sixteenth notes
notes.append(chromatic_scale.get(m.get('note') % 12))
return notesNow that we can select notes, and have replicated the encoding we just need to reverse our encoding functions. Here's the final code and output:
from mido import MidiFile
import mido as mido
chromatic_scale = {0: 'C', 1: 'C#', 2: 'D', 3: 'D#', 4: 'E', 5: 'F', 6: 'F#', 7: 'G', 8: 'G#', 9: 'A', 10: 'A#', 11: 'B'}
gmaj = {0: 'G', 1: 'A', 2: 'B', 3: 'C', 4: 'D', 5: 'E', 6: 'F#'}
def select_notes_from_file(midi_file):
midi_file = MidiFile(midi_file)
track = mido.merge_tracks(midi_file.tracks)
ticks_per_beat = midi_file.ticks_per_beat
notes = []
play_time = 0
for msg in track:
m = msg.dict()
play_time += m.get('time')
# each beat is a quarter note, we care about sixteenth notes
sixteenth = (play_time % ticks_per_beat)/(ticks_per_beat/4)
# Pick odd eighth notes
if (m.get('type') == 'note_on'
and m.get('velocity') != 0
and (sixteenth % 2) != 0):
notes.append(chromatic_scale.get(m.get('note') % 12))
return notes
def gmaj_to_sept(note_list):
gmaj_r = {v: k for k, v in gmaj.items()} # Reverse keys and values of gmaj dict
final = []
sept = ''
for note in note_list:
sept += str(gmaj_r.get(note))
if len(sept) == 3:
final.append(sept)
sept = ''
return final
def sept_to_dec(sept_list):
final = []
for sept in sept_list:
result = 0
digits = split(sept)
power = 0
while len(digits) != 0:
result += int(digits.pop()) * 7 ** power
power += 1
final.append(result)
return final
def dec_to_char(dec_list):
final = []
for dec in dec_list:
final.append(chr(dec))
return final
def split(word):
return [char for char in word]
notes = select_notes_from_file('Stegno.mid')
# Decoding
print('Selected notes by rhythm : {}'.format(', '.join(notes)))
sept = gmaj_to_sept(notes)
print('Base 7, 3 digits groups : {}'.format(', '.join(sept)))
decimal = sept_to_dec(sept)
print('Base 10 from Base 7 : {}'.format(', '.join(map(str, decimal))))
chars = dec_to_char(decimal)
print('ASCII TEXT : {}'.format(''.join(chars)))Selected notes by rhythm : A, B, B, A, D, B, A, E, G, A, E, C, B, C, D, A, D, G, B, B, E, B, B, C, A, G, G, A, B, D, A, F#, F#, B, A, C, A, F#, D, B, G, D, A, E, A, A, D, A, B, C, F#
Base 7, 3 digits groups : 122, 142, 150, 153, 234, 140, 225, 223, 100, 124, 166, 213, 164, 204, 151, 141, 236
Base 10 from Base 7 : 65, 79, 84, 87, 123, 77, 117, 115, 49, 67, 97, 108, 95, 102, 85, 78, 125
ASCII TEXT : AOTW{Mus1Cal_fUN}